Inscrivez-vous ou connectez-vous pour rejoindre votre communauté professionnelle.
Hollow steal pipes mor strength than others
For a given material, and given outer dimensions, the maximum strength is achieved if the rod is solid (not hollow), but this also takes a lot of weight.
For a given material, and given weight, the maximum strength is achieved by a hollow cylinder, that is of larger diameter than the solid rod of that weight.
Most of the strength of a cylinder comes from the outer portions. I think the contribution goes like the cube of the radial position. So, if you took a solid rod and drilled out a half the volume from the center, you do not lose half the strength. You only lose about10% of the strength, but you've saved on half the weight.
So, strength to weight ratio is better for a hollow pipe than a solid rod.
I guess a solid steel rod is stronger than a hollow steel pipe
Cause the second moment of a rod area is superior than that of a pipe
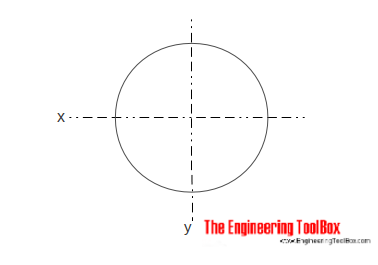
Ix = π r4 /4
= π d4 /64 (4)
where
r = radius
d = diameter
Iy = π r4 /4
= π d4 /64 (4b)
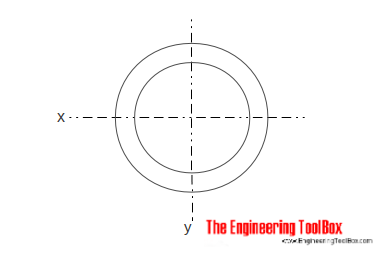
Ix = π (do4 - di4) /64 (5)
where
do = cylinder outside diameter
di = cylinder inside diameter
Iy = π (do4 - di4) /64
On a per pound basis, steel pipe is stronger in bending and is less likely to buckle in axial compression.
Hollow steel pipe
Agreed with the answer given by Mr. Alex Al Yazouri.
4down vote
http://www.physicsforums.com/archive/index.php/t-37701.html says
"Most of the strength of a cylinder comes from the outer portions. I think the contribution goes like the cube of the radial position. So, if you took a solid rod and drilled out a half the volume from the center, you do not lose half the strength. Strength to weight ratio is better for a hollow pipe than a solid rod."
The definition for the second moment of inertia Ic for a filled and hollow cylinder can be found onhttp://en.wikipedia.org/wiki/Second_moment_of_area:
Ic=∫∫Ay2dxdy=∫R0∫2π0r2dϕ rdr=πr44
The surface area of the filled cylinder is:
A=πr2
Compare filled and hollow cylinder of equal mass:
sc=IcA=r24 , cylinder with fractional internal radius ri=xro and x<1: sh=IhA=r4(1−x4)4r2(1−x2)=r2(1−x2)(1+x2)4(1−x2)=r2(1+x2)4>sc .
This means a hollow cylinder is stronger than a rod of equal mass and the same material. A hollow cylinder with a bigger inside diameter is better. In the limit x→1 the hollow cylinder is twice as strong. Note that this limit isn't physically viable as it would be an cylinder with infinite radius and infinitesimally thin wall. However it is useful to define the upper limit of the second moment of inertia. I didn't expect the increase in strength only a factor of two.
Depends on steel quality, diameter, and thickness of the pipe and solis pipe. With these three you can determine the mechanical values (A, W, I) and see what is stronger and why.
Hollow steel pipe is stronger if the length and weight are the same because it is harder to strain a denser object that less dense.
solid rod is stronger than hollow rod in all of load cases
but we are Engineers , we use stronger and economic so if load is axial load we use solid rod as it more stronger than hollow rod but if shear load we use hollow in safe side as it more economic
